Marjon Tas

Global description of the circle frame

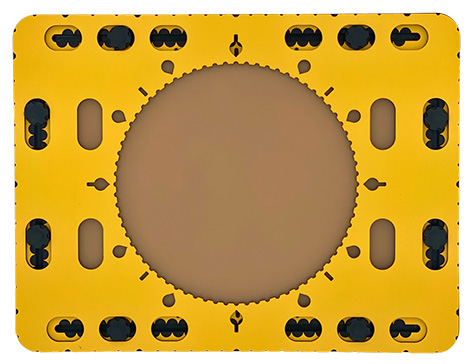
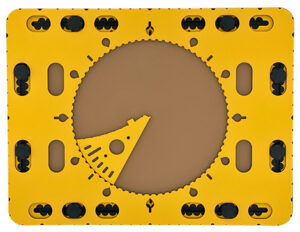
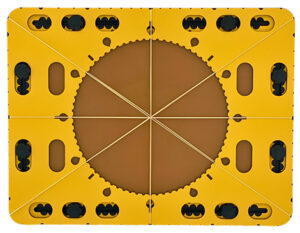
The actual frame of the CircleFrame has similar dimensions as the TactiPad (28 cm by 37 cm). The thickness of the frame is four millimetres. Near the outer edge of the frame oval holes can be found to place the frame in one of three positions on the TactiPad. For an a-centric position, two additional holes are present at both sides near the circle.

To description section
To manual section

Detailed description

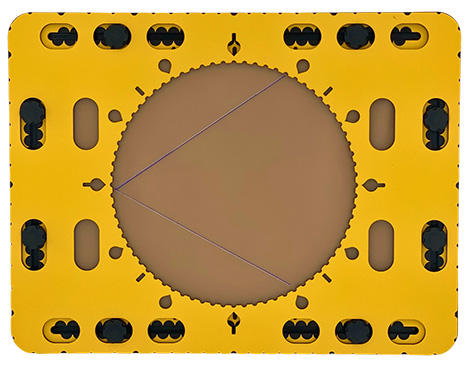
In the middle of the CircleFrame is a large circle cut out with a diameter of 20 centimetres. This is the actual drawing area. Along the edge of this circle medium sized indents every five degrees are provided, which form a large protractor. Around the outside of the circle there are extra markings: a teardrop shape for every 30 degrees, a line for every 45 degrees, or a combination of both where they coincide at 0, 90, 180 and 270 degrees. Indentations are placed around the outside of the circle frame in which rubber bands can be attached. These indentations are positioned at every 10 degrees seen from the centre of the circle. Slightly wider indents make the positions for 0, 30, 45, 60, 90 degrees, etc. easily recognizable

Circle frame manual

To description section

Storage of the CircleFrame

The frame of the CircleFrame will sit or can sit already on top of the drawing board for storage. The additional tools for the CircleFrame have their place in the paper pocket.

Preparing the drawing board

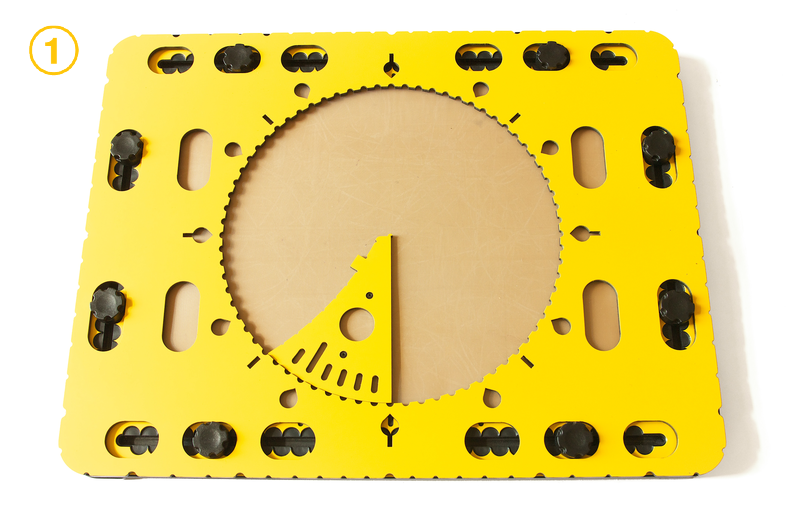
Place the knobs of the TactiPad five centimetres away from the corners. The CircleFrame can be placed n three different positions: in the middle of the drawing board with the edges coinciding with those of the TactiPad, or in two positions left and right of the drawing board’s centre when in landscape orientation. Make sure the eight oval holes near the outer edge of the CircleFrame fit all over a knob.

Because the circle is exactly in the middle of the circle frame, the frame is horizontally and vertically symmetrical. Mounting the CircleFrame on the TactiPad therefore needs no consideration.

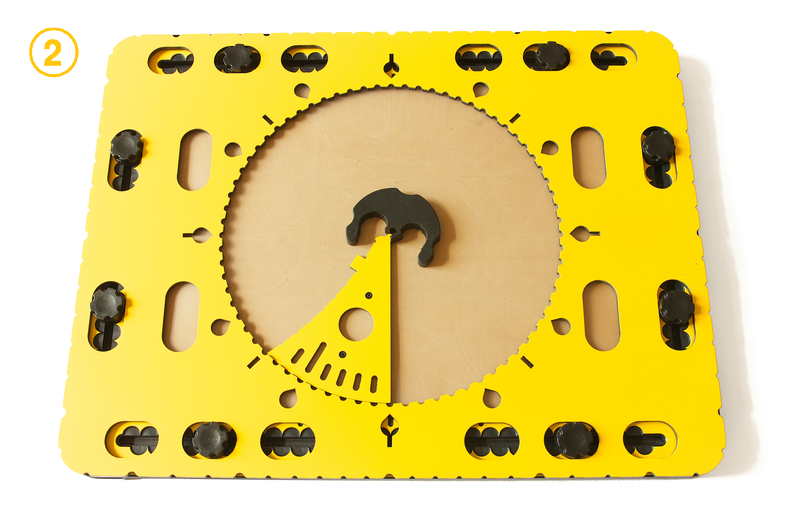
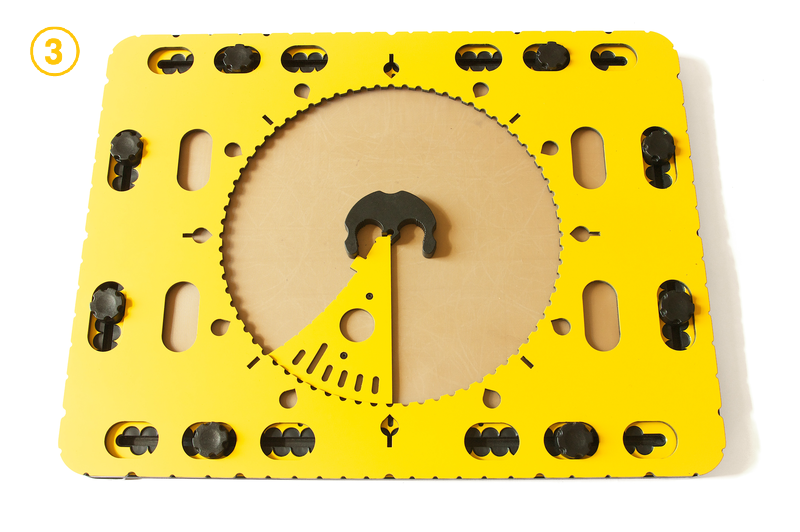
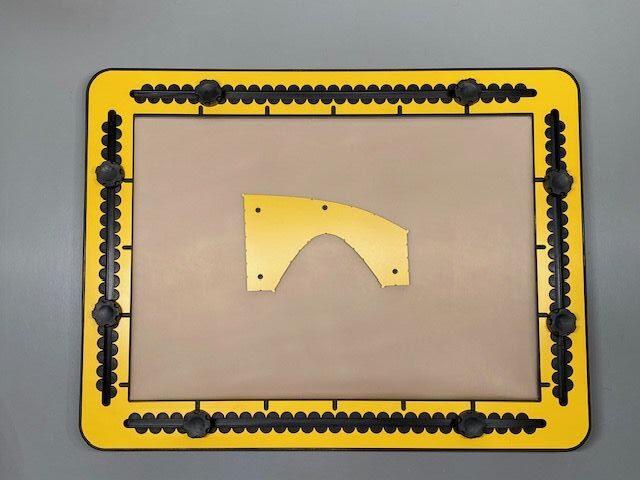
Inserting the tools in the circle

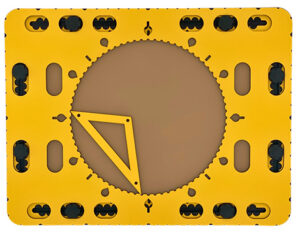
The wedge tool, the 468triangle or the zigzag triangle can rotate freely over 360 degrees within the circle. The drawing tools preferably are aligned with the degree markings along the circle.

For detailed descriptions and manuals of related tools see:

03 Zigzag triangle
04 468Triangle
05 Wedge tool
06 More ideas

 Back to description (top)

Global description

The size of the zigzag triangle is such so that it fits exactly inside the 20 centimetres diameter of the circle.The three sides of the body of the triangle all have a different pattern. The middle section of the triangle is open. The inner sides have the same patterns at a smaller scale as along the outside. The zigzag triangle is also called a ‘Christmas tree’.

To description section
To manual section

Detailed description

The corners of the triangle are rounded. One corner has an alignment indentation to align the triangle with the degree markings along the edge of the circle. The side opposite the indented corner is straight. Another one provides a smooth wave pattern, the final one has a zigzag pattern. Along the inner and outer side of the straight side two small indents indicate the length of a line segment to construct an hexagon. In the straight side oval holes can be found to hold a pen while rotating the triangle. The holes are placed to obtain curves at four, five, six, seven, eight or nine centimetres relative to the centre of the circle. Pushpin markers are placed near the corners.

Zigzag triangle manual

To description section

Preparing the TactiPad

Place all eight knobs of the TactiPad approximately five centimetres away from the corners. Place the circle frame on top of the TactiPad.

Using the zigzag triangle

The zigzag triangle fits exactly inside the circle with the rounded corners nearly touching the circle edge. It can rotate freely. Place your pointing finger on the alignment indent and align the triangle at 12 o’clock. Now you can create an equilateral triangle inside the circle. Start with the indented corner at 12o’clock and draw along the straight outside of the triangle. Rotate the tool over 120 degrees clockwise (which is at 4 o’clock) and draw the line. Rotate once more over 120 degrees (which is at 8 o’clock).

Hexagon in the circle

Align the indented corner at any degree indication and draw a line only between the two indents along the inner or outer straight side. Rotate the triangle over 60 degrees and repeat the draw. In total four more rotations are required to finish the shape.

Repetitive patterns; Mandala type of figures

By tracing segments of the sides and/or combining patterns intriguing drawings will emerge. Whatever you do, the result is fascinating.

Oval holes to create arches

The holes in the straight side of the triangle can hold a pen. Rotate the triangle holding the pen. Arches with a length depending on the amount of rotation and the selected radius will occur.

 Back to top of page

Global description

The 468triangle is an open triangular shaped frame with a width of one centimetre. The number 468 refers to the steps required for the triangle to return on the exact same position in the circle; four, six or eight steps respectively. Beyond this it serves as a template for ‘home made’ templates.

To description section
To manual section

Detailed description

The corners of the triangle are pointy so they fit in the indents at the edge of the circle of the framework. The length of the sides are designed so they exactly span an arc of 45, 60 or 90 degrees, seen from the centre of the circle. Pushpin markers are located at the top surface.

468Triangle manual

To description section

Square, hexagon and octagon

The 468triangle can have its starting position anywhere in the circle. Tracing along the longest inner side of the triangle results in a square. Tracing the shortest side gives an octagon. The third one an Hexagon.

Home made templates

The 468triangle can serve as an example to create your own drawing templates out of cardboard or an other sturdy material. By giving one side of the template a length similar to one of the triangle sides, it will fit by rotating eight, six or four times in the full 360 degrees of the CircleFrame.

Your template

Cut out an irregular shape from the centre of your template. Place the template against the edge of the circle and trace the inner contour. Depending on the chosen length, rotate the template 45, 60 or 90 degrees to obtain a symmetrical pattern. Recurrence and/or partial overlap will give your drawing an unexpected appeal.

 Back to top of page

Detailed description

The wedge-shaped drawing tool has a rounded edge that matches the curve of the circle in the CircleFrame. This rounded side covers an arc of 45 degrees. Near the rounded edge grooves per six degrees (one minute) are located. A little further away from the edge a hole is present. Two sides of this tool end in a flattened point. One of these sides is straight and ends in a very small point that serves as a pen blocker. This pen blocker also exactly coincides with the centre of the circle. The straight side forms the radius for the circle. The other side of the wedge is slightly curved inward. This side has small indents at every centimetre. The small block between the indents at 2 and 3 centimetre is used to position the compass base.

To description section
To manual section

Wedge tool manual

To description section

Make sure the CircleFrame is placed properly on the TactiPad.

Drawing pie charts

The wedge tool can be used to draw pie charts, which are often used to present statistic information in fields such as mathematics, economics and geography. The quantity that each sector represents corresponds with an arc of a certain number of degrees. By determining the angle between two radii on the inside of the circle, you can draw the pie chart very accurately. Keep the tool in place with one or two fingers in the hole in the tool and press it against the edge of the circle.

 Many other mathematical concepts in which degrees and angles play a key role, such as vectors or polar and trigonometric functions, can be explained and drawn as well using the CircleFrame. Centring the compasses in the CircleFrame. Another function of the wedge tool is to position the compass in the centre of the CircleFrame. You can achieve this by placing the wedge tool anywhere against the edge of the circle with the compass base against the flattened tip of the wedge. The centre of the compass base now hooks around the pen blocker at the tip of the wedge tool.

By slightly turning the base counter clockwise, it will catch the small protruding block on the curved side of the wedge. This way, the compass base is exactly in the middle of the circle. By removing the wedge tool and placing the compass arm into the base, you can draw a circle precisely in the centre of the CircleFrame.

Figure 2. Position the wedge tool along the edge of the circle (step 1), position the compass base and turn it so it rests against the wedge tool (steps 2 and 3) and remove the wedge tool (step 4). The compass is now perfectly positioned in the centre.

Drawing the hands of a clock

Around the circle of the CircleFrame, multiples of 30 degrees are indicated by drop-shaped indentations. These make up for the twelve hours of the clock. To draw a clock hand, you can use the wedge tool. Along the rounded side you will find grooves at an angle corresponding to 1 minute, or 6 degrees. The longer groove of 2 centimetre indicates the position of 5 minutes or 1 hour later. After placing the wedge tool with the rounded side against the edge of the circle and aligning the straight side with one of the hour indications, you can set the minute and draw the hands of the clock as radii and have it set at any desired time.

Rubber bands spanning the diameter

Indentations are placed around the outside of the circle frame in which rubber bands can be attached. These indentations are positioned at every 10 degrees seen from the centre of the circle. Slightly wider indents make the positions for 0, 30, 45, 60, 90 degrees, etc. easily recognizable. When spanning to opposing indentations with a rubber band, they meet the centre, forming also the diameter of the circle.
It also exactly coincides with the degree indications along the circle’s edge.

Compass: circles in a circle

You can also combine the compass with the edge of the circle. The indents per five degrees are semi-circular and have the same diameter as the needle of the compass. Place the compass foot partly on the edge so the needle of the compass fits in an indentation of the CircleFrame. Set the desired radius for the circle to be drawn. The set radius for the circle determines the length of the curve that can be created. The centre of the arcs are now exactly on the edge of the frame. By drawing multiple arcs along the edge of the circle, you can create intriguing repetitive geometric patterns.

Fantasy

As an example set the radius at 10 centimetres. Place the compass base at 12 o’clock and draw the maximum possible curve. Place the base at 3, 6 and 9 o’clock respectively and create the curves as well. Trace the circle’s contour and remove the circle frame. The curves will cross in the middle of the circle.